Complementary Slackness Theorem
Complementary Slackness Theorem - Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. Let p and d denote the primal and dual linear program (in standard form) respectively. One thing we can use complementary slackness for is to verify claims about optimal solutions. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. The complementary slackness1 theorems state. Say someone tells us that \(x_1^* =.
Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Let p and d denote the primal and dual linear program (in standard form) respectively. The complementary slackness1 theorems state. One thing we can use complementary slackness for is to verify claims about optimal solutions. Say someone tells us that \(x_1^* =. Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and.
Say someone tells us that \(x_1^* =. One thing we can use complementary slackness for is to verify claims about optimal solutions. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. The complementary slackness1 theorems state. Let p and d denote the primal and dual linear program (in standard form) respectively.
The Complementary Slackness Theorem (explained with an example dual LP
Let p and d denote the primal and dual linear program (in standard form) respectively. Say someone tells us that \(x_1^* =. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. Theorem (complementary slackness) let x be a feasible solution to the primal and y be.
Complementary slackness and farkas lemmaa
Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. The complementary slackness1 theorems state. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. Let p and d.
V412. Linear Programming. The Complementary Slackness Theorem. part 2
Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. The complementary slackness1 theorems state. Let p and d denote the primal and dual linear program (in standard form) respectively. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in.
complementary slackness theorem with examples YouTube
Let p and d denote the primal and dual linear program (in standard form) respectively. Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in.
1 Complementary Slackness YouTube
Let p and d denote the primal and dual linear program (in standard form) respectively. Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. Let x be a primal feasible solution and let u be a dual feasible solution such that.
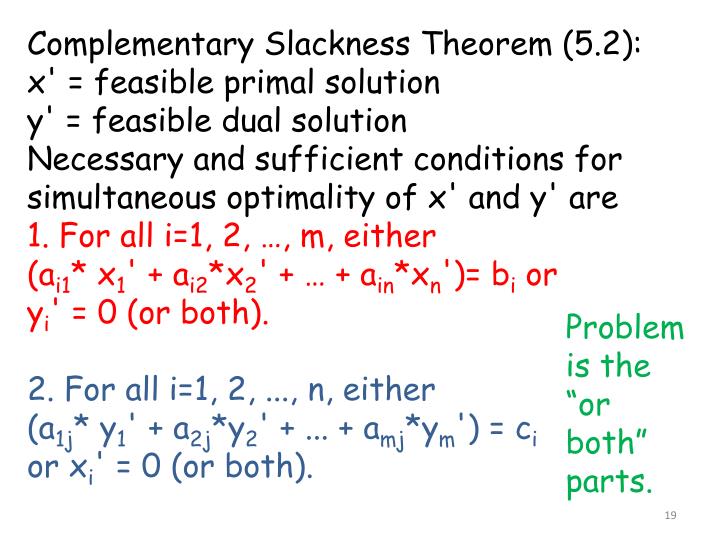
PPT Summary of complementary slackness 1 . If x i ≠ 0, i = 1, 2
Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. Let p and d denote the primal and dual.
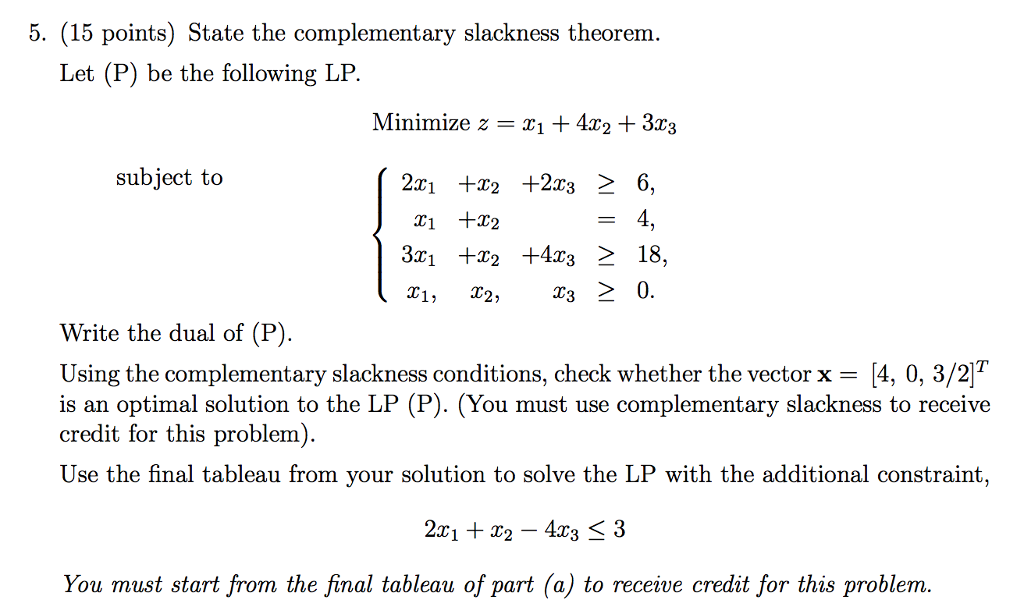
5. (15 points) State the complementary slackness
Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Let p and d denote the primal and dual linear program (in standard form) respectively. Say someone tells us that \(x_1^* =. Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to.
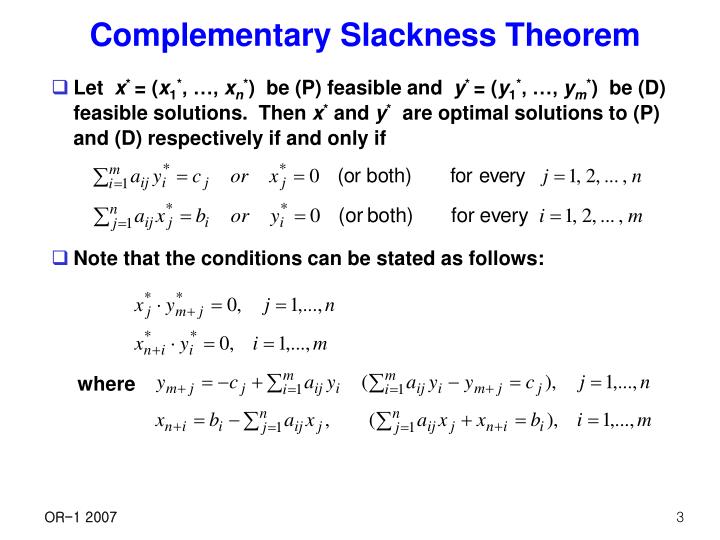
PPT Relationship between (P) & (D) PowerPoint Presentation ID3895569
The complementary slackness1 theorems state. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. One thing we can.
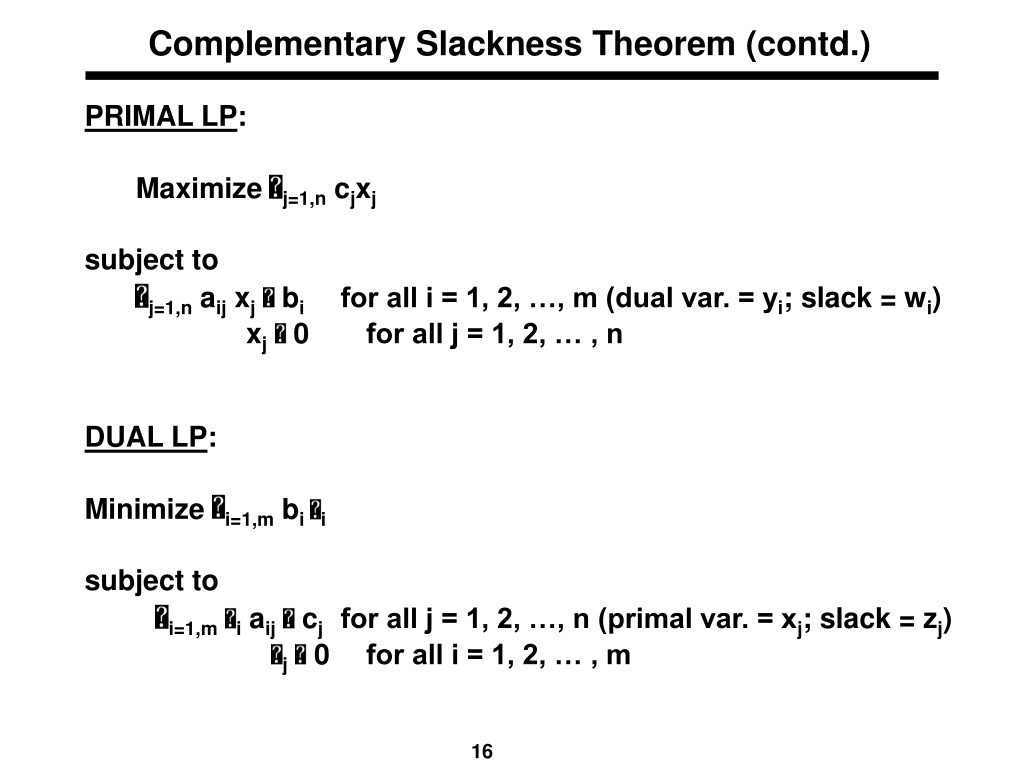
PPT DUALITY THEORY PowerPoint Presentation, free download ID9471038
Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. The complementary slackness1 theorems state. Let p and d.
V411. Linear Programming. The Complementary Slackness Theorem. YouTube
Let p and d denote the primal and dual linear program (in standard form) respectively. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Theorem (complementary slackness).
Let P And D Denote The Primal And Dual Linear Program (In Standard Form) Respectively.
Theorem (complementary slackness) let x be a feasible solution to the primal and y be a feasible solution to the dual where primal max c x ax b. Let x be a primal feasible solution and let u be a dual feasible solution such that complementary slackness holds between x and. One thing we can use complementary slackness for is to verify claims about optimal solutions. Say someone tells us that \(x_1^* =.
The Complementary Slackness1 Theorems State.
Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming.